
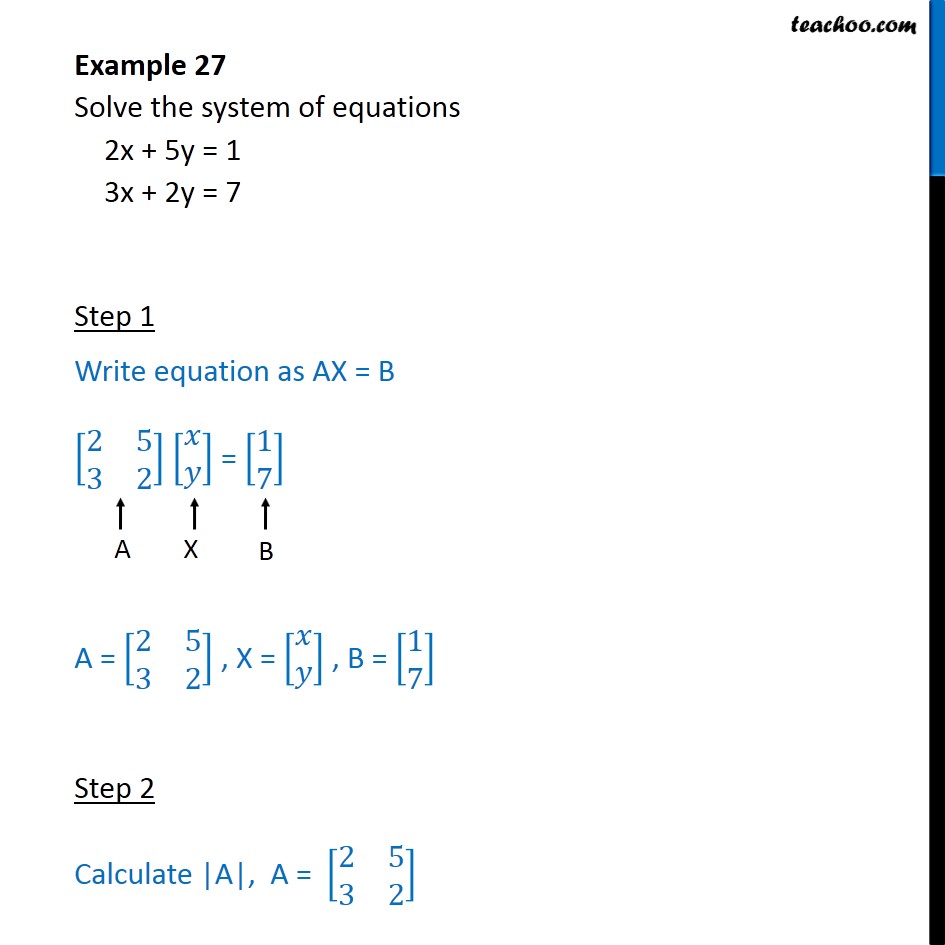
So we’ll need to multiply one of the equations by some number such that by combining the resulting equation with one of the other two equations, we’ll be able to eliminate a variable. None of the terms with the same variable have the same coefficient (or coefficients that are equal in absolute value but opposite in sign). Use any method to solve the system of equations. Now choose one of the three original equations, and plug in ?-1? for ?x? and ?-4? for ?z?, and then solve for ?y?. If we add these two equations, we can eliminate the variable ?z?, and then solve for ?x?.Ĭhoose one of the new equations, and plug in ?-1? for ?x?, and then solve for ?z?. The coefficients of ?z? in our two new equations are ?-2? and ?2?, respectively. You might have also noticed that the coefficients of ?y? in equations and are ?-5? and ?5?, respectively, so we can add these two equations to get another equation in only the variables ?x? and ?z?. Remove parentheses and combine like terms.

If we add these two equations, the ?y? terms will cancel (we’ll eliminate the variable ?y?) and we’ll get an equation in only the variables ?x? and ?z?.
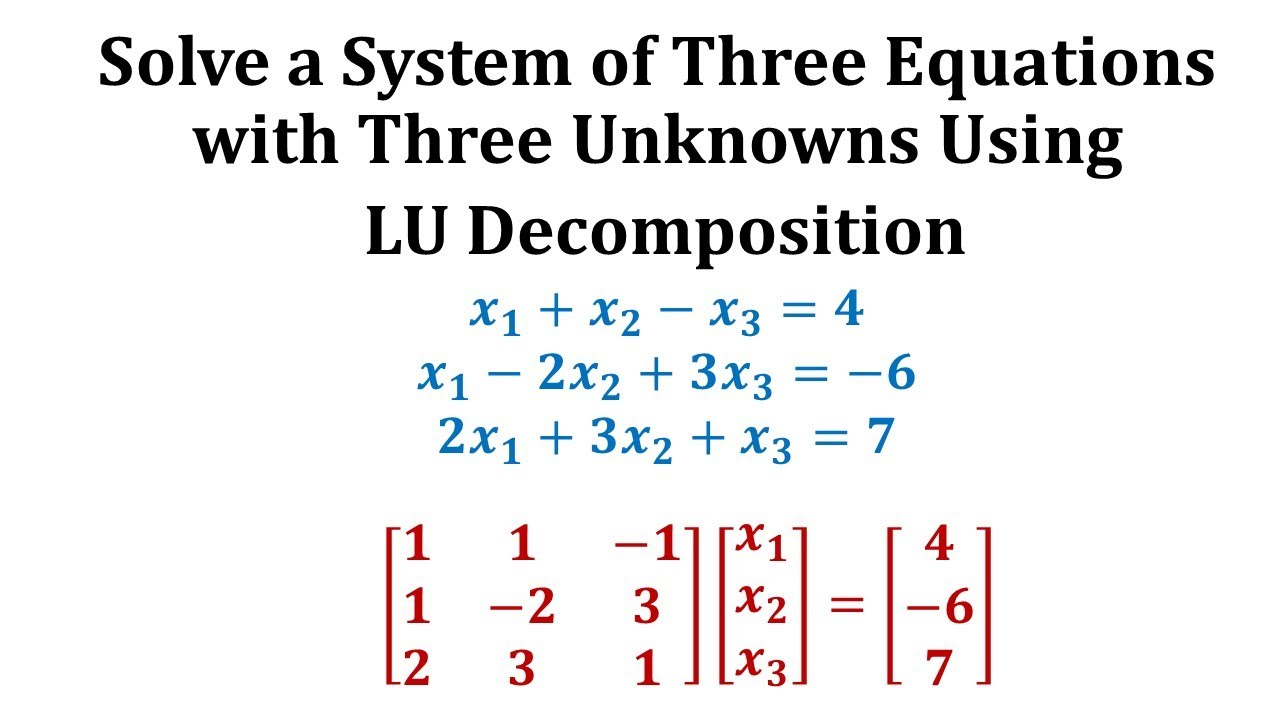
Notice that the coefficients of ?y? in equations and are ?-5? and ?5?, respectively.


 0 kommentar(er)
0 kommentar(er)
